top of page

Homeschooled First Grader (Braintree, MA)
Mathematics
The First Surprise
November 10, 2019 (Kindergarten)
This is the story of how one Sunday morning when he was around five and a half year old Mortaza first surprised me by showing an unusual interest and aptitude in math.
Read the story here
The Empty Block
Spring 2020 (Kindergarten)
How Mortaza found a way to calculate the square of numbers based on the square of smaller numbers.
Read the story here
The Reason Behind Division
August 6 - 2020 (Kindergarten)
Mortaza's Explanation of why 630 divided by 70 is equal to 9.
Read the story here
Infinity
September 22 - 2020 (1st grade)
Mortaza has always been fascinated by extremely large numbers and of course the concept of infinity. In this video, Morteza explanations (in Farsi) why the product of any number by infinity will be infinity. This seems to be something that he found on his own and he uses his own notation. He just learned the infinity sign from us.
Adding Two and Three-Digit Numbers (and more)
October 23 - 2020 (1st grade)
Mortaza can add two- and three-digit numbers in his mind. As an indoor physical exercise he jumps on a mattress and counts the number of strips on the mattress that he jumps on. He repeats this and adds the length of jumps to achieve certain distance.
Prime Numbers and Prime Factors
December 5 and 11 - 2020 (1st grade)
Mortaza understands the concept of prime numbers and can identify the prime factors of numbers.
Equations and Systems of Equations
December 13 and 23 - 2020 (1st grade)
Mortaza can solve simple equations and systems of equations, again in his mind.
Ratios, Fractions, and More
December 15 to 22 - 2020 (1st grade)
Here is Mortaza's practice with ratios, fractions and simple equations.
Pie Chart
December 23 - 2020 (1st grade)
I don't know where he learnt to draw a pie chart. He didn't know it was called a pie chart. Later, he told me that these are the amounts of minerals absorbed by plants through their roots. I am not sure if these values are real or has been made up by him.
Popsicle Riddles
Started in September 23, 2020 (1st grade)
These are math riddles designed by Mortaza using popsicle sticks or pens.
Bedtime Challenges
Started in January 18, 2021 (1st grade)
These are math problems through which Mortaza and I challenged each other before his bedtime.
How Old is Mortaza?
February 4, 2021 (1st grade)
With some help from me, Mortaza calculated how many days, hours, and second he has lived.
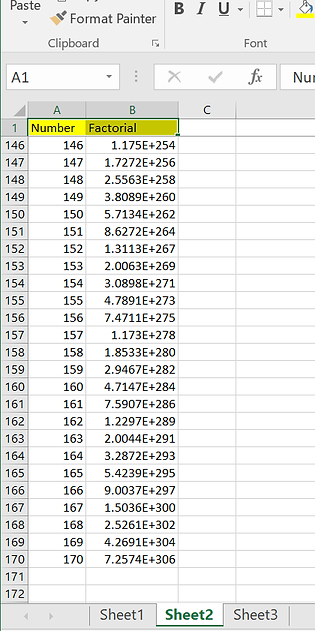
Factorial and the Size of the Universe?
February 15, 2021 (1st grade)
Mortaza already knew the definition of factorial. But on the afternoon of February 15, for some reasons which was unknown to me, I found Mortaza with a renewed and vigorous interest in factorial of numbers. He was particularly interested in the factorial of large numbers. So, I showed him how to calculate the factorial of numbers using a scientific calculator. I also showed him how we could do the calculations in Excel. The Excel could calculate the factorial of numbers up to 170. Naturally, the result was a humongous number.
I told Mortaza this could be larger than the size of the universe. His answer was very interesting: "of course, if you assume that the universe is not infinite!"
He then asked why we couldn't calculate the factorial of decimal numbers. He proposed a method of his one. Of course, it was not consistent with the formal definition.
4.5! = 4.5 * 4.4 * ... * 0.2 * 0.1
_b.png)
Logarithmic Riddles
February 26, 2021 (1st grade)
This is the story of how Mortaza surprised us by his unexpected understanding of logarithm.
Impossible Equation
March 25, 2021 (1st grade)
Again, in an attempt to win more computer game time, Mortaza wrote me this equation and aske me to find X.
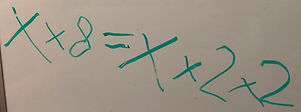.jpg)
I had a suspicion about what he was up to. So, I said, "The equation doesn't have a finite answer." To which he replied with a wide smile, "X is infinity." I told him that X cannot be infinity but can approach it. He used the right terms when he told his mother about it later.
Mathematical Induction
March 26, 2021 (1st grade)
Mortaza came to my office and told me that he needed to use Excel. I let him. He created the file below. As you can see in the second raw, each number is the sum of the two previous numbers. The first row shows 2 to the power of the numbers in the second row. I asked him what it was. He said it was about "induction". He explained he had created a repeating pattern like what we could have in "induction". He said he had learned about induction in this video.
Later on, I asked him what induction was (the video). Although his definition was not completely accurate, it seems that he has gotten the gist of the concept.
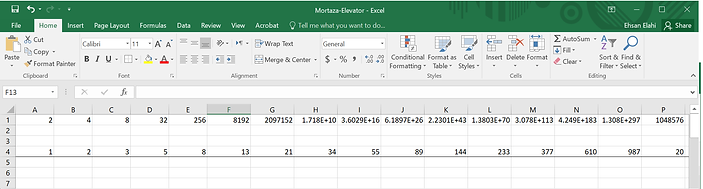
How to Create Numbers with a Repeating Pattern
April 3, 2021 (1st grade)
Mortaza came to my office and asked me to let him work with the calculator on my computer. I left the room and let him do it. When I came back, he told me if I knew how make the number 755,755 from 755. He said, we had to multiply 755 by 1001 to get 755,755. He said that he had learned it from this video. Then he generalized the concept and said if you wanted to repeat any number you had to multiply it by a number with the form of 1001 with the the number of digits equal to one more than the number of digits of the original number. He showed me why this worked.

Numbers Divisible by three and seven
May 27, 2021 (1st grade)
My wife told me that, the previous night, Mortaza had explained to her why the numbers whos sum of digits were divisible by three, were divisible by three themselves. I asked Mortaza to explain it to me too. He immediately went to the whiteboard to explain it. I managed to record him. He then added that the same reasoning explains the divisibility by 9. I didn't know that the rule of divisibility by 3 and 9 had such an elegant proof.
When Mortaza saw my interest in topic, he continued to explain to me the rule of divisibility by 7.
Multiplying number that have factors of 10 in them
May 27, 2021 (1st grade)
While reading "The Life of Fred", which is Mortaza's math textbook, at some point we had to multiply 1500 and 700.
Mortaza told me he has a general rule for multiplying such numbers and he wrote on the screen the result of X*Y. In this figure Ex and Ey are the number of zero digits on the right side of the X and Y, and Fx and Fy hold the remaining digits. I was impressed by his ability to generalize this simple fact and present them with mathematical terms.
.jpg)
Another Riddle
Jan 27, 2021 (1st grade)
Mortaza asked me to play another game of 3D chess with him. I told him that I would do it if he gave me a difficult riddle. He then went to the whiteboard and wrote this:
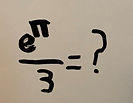.jpg)
I said I didn't know the answer. He replied the answer was almost 7.666..... In answer to my question of how he knew, he said, "I calculated e to the power of pi a few days ago with a calculator and it was a little bit more than 23. Since 23/3 is 7.6666... then the answer to the riddle should be almost this number.
Difficult Questions
August 2022 (2nd grade)
We were on a week-long family trip to Karbala. Mortaza, his cousin Noora (15 years old), and I were in the room. To calm down the hyperactive Mortaza, I suggested let's play a game of taking turns asking each other difficult questions. The person with the most correct answers would be the winner. Mortaza asked the first question: "What is e to the power of pi if we round it to the nearest whole number?". I forgot that Mortaza had asked me this question more than a year ago. Naturally, none of us answered it. So, he said 23. Next, Noora asked: "What countries are part of two continents?" Mortaza answered Turkey and Russia. Next, I asked: "What are the largest states in the US?" Mortaza and Noora, together (after a few iterations), answered Texas, Alaska, and California. Then it was Mortaza's turn again. He asked: "What is the sum of the volumes of all unit spheres in even dimensions?". This was another of Mortaza's questions that I didn't understand the meaning of the question. When Mortaza didn't hear any answer from us, he smiled and said, "The answer is e to the power of pi." Later on, I watched this video to understand the meaning of his question.
bottom of page
.jpg)
.jpg)







_bmp.png)

_bmp.png)